
The Geodynamics 101 series serves to showcase the diversity of research topics and methods in the geodynamics community in an understandable manner. We welcome all researchers – PhD students to Professors – to introduce their area of expertise in a lighthearted, entertaining manner and touch upon some of the outstanding questions and problems related to their fields. This month Juliane Dannberg from Colorado State University, discusses the influence of grain size and why it is important to consider it in numerical models. Do you want to talk about your research? Contact us!

Juliane Dannberg
When I started my PhD on geodynamic modelling, I was not aware that the size of mineral grains was something I might need to consider in my simulations. To me, grain size was something that sedimentologists need to describe rocks, and not something I had to deal with in my computations. In all the modelling papers I had read, if the mineral grain size was even mentioned, it was always assumed to be constant. However, it turns out that these tiny grains can have huge effects.
I first heard about the importance of grain size in a series of lectures given by Uli Faul when I participated in the CIDER summer program in 2014 (in case you’re interested, the lectures were recorded, and are available here and here). Primarily, I learned that for diffusion creep – the deformation mechanism people predominantly use in convection models – the viscosity does not only depend on temperature, but is also strongly controlled by the grain size, and that this grain size varies both in space and in time.
This made me think. If grain size in the mantle changes by several orders of magnitude, and the viscosity scales with the grain size to the power of 3, didn’t that mean that grain size variations could cause huge variations in viscosity that we do not account for in our models? Shouldn’t that have a major effect on mantle dynamics, and on the evolution of mantle plumes and subduction zones? How large are the errors we make by not including this effect? I was perplexed that there was such a major control on viscosity I had not thought about before, and wanted to look into this further myself.
Luckily, the multidisciplinary nature of CIDER meant that there were a number of people who could help me answer my questions. I teamed up with other participants1 interested in the topic, and from them I learned a lot about deformation in the Earth’s mantle.
How does the mantle deform?
For most of the mantle, the two important deformation mechanisms are diffusion and dislocation creep. In diffusion creep, single defects (or vacancies) – where an atom is missing in the lattice of the crystal – move through this lattice and the crystal deforms. For this type of deformation, the strain rate is generally proportional to the stress, which means that the viscosity does not depend on the strain rate. Many global mantle convection models use this kind of rheology, because it is thought to be dominant in the lower mantle, and also because it means that the problems described using this rheology are usually linear, which makes them easier to solve numerically. Furthermore, this is also the deformation mechanism that depends on the grain size. Usually, it is assumed that the viscosity scales with the grain size to the power of 3:
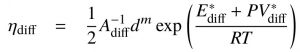
where ndiff is the diffusion creep viscosity, d is the grain size, m=3, T is the temperature, P is the pressure, and Adiff, E*diff, V*diff and R are constants.
However, if the stress is high (or the grain size is large, Figure 1), dislocation creep is the dominant deformation mechanism. In dislocation creep, linear defects – so-called dislocations – move through the crystal and cause deformation. In this regime, the viscosity depends on the strain rate, but not on grain size. Dislocation creep is generally assumed to be the dominant deformation mechanism in the upper mantle.
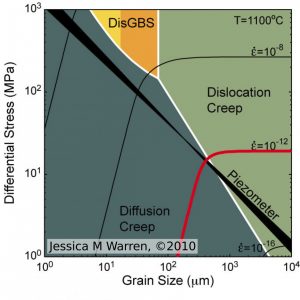
Figure 1: Deformation mechanisms in olivine
Why do grains grow or shrink?
In general, from an energy standpoint, larger crystals are more stable than smaller crystals, and so crystals tend to grow over time in a process called Ostwald ripening. The smaller the grains are, and the higher the temperature, the faster the grains grow. One the other hand, the propagation of dislocations through the grains causes so-called dynamic recrystallization, which reduces the grain size if the rock deforms due to dislocation creep. This means that there are always the competing mechanisms of grain growth and grain size reduction, and their balance depends on the dominance of either of the two deformation mechanisms described above – diffusion or dislocation creep:
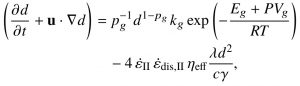
The left-hand side of the equation describes the change of the grain size d over time, the first term on the right-hand side is grain growth (depending on grain size and temperature), the second term describes grain size reduction (depending the strain rate, the stress, and also on the grain size itself). The parameters Pg, kg, Eg, Vg, R, λ, c and γ are all constants.
If the flow field does not change, grains will evolve towards an equilibrium grain size, balancing grain growth and grain size reduction. In addition, the grain size may change when minerals cross a phase transition. If the mineral composition does not change upon crossing a phase transition (a polymorphic phase transition such as olivine–wadsleyite), there is almost no effect on grain size. But if the composition of the mineral that is stable after crossing the transition is different from the one before, the mineral breaks down, and the grain size is reduced, probably to the micrometer-scale [Solomatov and Reese, 2008].
And what does that mean for the dynamics of the Earth?
As there is a complex interaction between grain size evolution, mantle rheology and the deformation in the mantle, it is not straightforward to predict how an evolving grain size changes mantle dynamics. But it turned out that there had been a number of modelling studies investigating this effect. And they indeed found that grain size evolution may substantially influence the onset and dynamics of convection [Hall and Parmentier, 2003], the shape of mantle plumes [Korenaga, 2005], mixing of chemical heterogeneities [Solomatov and Reese, 2008], the seismic structure of the mantle [Behn et al., 2009], and the convection regime and thermal history of terrestrial planets [Rozel, 2012].
The long way to a working model…
But even knowing all of these things, it was still a long way to implement these mechanisms in a geodynamic modelling code, testing and debugging the implementation, and applying it to convection in the Earth. There were several reasons for that:
Firstly, large viscosity contrasts are already a problem for most solvers we use in our codes, and the strong dependence of viscosity on grain size means that viscosity varies by several orders of magnitude over a very small length scale in the model.
Secondly, considering an evolving grain size makes the problem we want to solve strongly nonlinear: Already in models with a diffusion–dislocation composite rheology and a constant grain size, the viscosity – which is needed to calculate the solution for the velocity – depends on the strain rate, making the momentum conservation equation nonlinear. But an evolving grain size introduces an additional nonlinearity: The viscosity now also depends (nonlinearly) on the grain size, whose evolution in turn depends on the velocity field. In terms of dynamics, this means that there is now another mechanism that can localize deformation. If the strain rate is large, the grain size is reduced due to dynamic recrystallization (as described above). A smaller grain size means a lower viscosity, which again enables a larger strain rate. Due to this feedback loop, velocities can become very high, up to several meters per year, which severely limits the time step size of a numerical model.
Finally, the equation (2) that describes grain size evolution is an ordinary differential equation in itself, and the time scales of grain growth and grain size reduction can be much smaller than changes in the flow field in the mantle. So, in order to model grain size evolution and mantle convection together, one has to come up with a way to separate these scales, and use a different (and probably much smaller) time step to compute how the grain size evolves. I remember that at one point, our models generated mineral grains the size of kilometers (whereas the grain sizes we expect in the mantle are on the order of millimeters), because we had not chosen the time step size properly. And on countless occasions, the code would just crash, because the problem was so nonlinear that a small change in just one parameter or a solution variable had such a large impact that material properties, velocities and pressures went outside of the range of what was physically reasonable.
However, after a lot of debugging, we could finally investigate how an evolving grain size would influence mantle dynamics. But see for yourself below. In an example from our models, plumes become much thinner when reaching the upper mantle, and cause much more vigorous small-scale convection when they interact with the lithosphere. Slabs bend rather than thicken, and accumulate as piles at the core-mantle boundary.
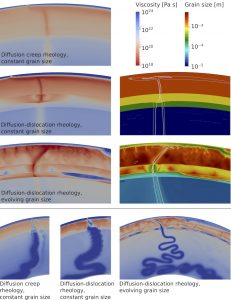
Figure 2: Comparison of plumes and slabs in models with and without grain size evolution. Modified from Dannberg et al., 2017
Of course, there are also many other areas where grain size evolution is important, and many recent studies are concerned with the influence of grain size on the Earth’s dynamic evolution. Dave Bercovici and his collaborators found that grain evolution and damage mechanisms may be a key factor for the onset of plate tectonics [e.g. Bercovici and Ricard, 2014, 2016]: Grain size reduction in shear zones could make them weak enough to for subduction initiation. The evolution of grain size may also be a major factor for focusing of melt to mid-ocean ridges [Turner et al., 2017], as it influences how fast the solid matrix can dilate and compact to let melt flow in and out. And if the Large Low Shear Velocity Provinces at the core-mantle boundary are indeed piles of hot material that are stable on long time scales, mineral grains would have a long time to grow and may play a crucial role for pile stability [Schierjott et al., 2017].
So if you do not include grain size evolution in your geodynamic models – which in many cases is just not feasible to do – I hope that you now have a better feeling for how that may affect your model results.
1The other researchers in my CIDER group were Zach Eilon, Ulrich Faul, Rene Gassmöller, Raj Moulik and Bob Myhill. I learned a lot about grain size in the mantle in particular from Bob Myhill and Ulrich Faul; I developed the geodynamic models together with Rene Gassmöller, and Zach Eilon and Raj Moulik investigated how the evolving grain size predicted by our models would influence seismic observations.
References: Solomatov, V. S., & Reese, C. C. (2008). Grain size variations in the Earth's mantle and the evolution of primordial chemical heterogeneities. Journal of Geophysical Research: Solid Earth, 113(B7). Hall, C. E., & Parmentier, E. M. (2003). Influence of grain size evolution on convective instability. Geochemistry, Geophysics, Geosystems, 4(3). Korenaga, J. (2005). Firm mantle plumes and the nature of the core–mantle boundary region. Earth and Planetary Science Letters, 232(1), 29-37. Behn, M. D., Hirth, G., & Elsenbeck, J. R. (2009). Implications of grain size evolution on the seismic structure of the oceanic upper mantle. Earth and Planetary Science Letters, 282(1), 178-189. Rozel, A. (2012). Impact of grain size on the convection of terrestrial planets. Geochemistry, Geophysics, Geosystems, 13(10). Dannberg, J., Eilon, Z., Faul, U., Gassmöller, R., Moulik, P., & Myhill, R. (2017). The importance of grain size to mantle dynamics and seismological observations. Geochemistry, Geophysics, Geosystems. Bercovici, D., & Ricard, Y. (2014). Plate tectonics, damage and inheritance. Nature, 508(7497), 513-516. Bercovici, D., & Ricard, Y. (2016). Grain-damage hysteresis and plate tectonic states. Physics of the Earth and Planetary Interiors, 253, 31-47. Turner, A. J., Katz, R. F., Behn, M. D., & Keller, T. (2017). Magmatic focusing to mid-ocean ridges: the role of grain size variability and non-Newtonian viscosity. arXiv preprint arXiv:1706.00609. Schierjott, J., Rozel, A., & Tackley, P. (2017, April). Toward unraveling a secret of the lower mantle: Detecting and characterizing piles using a grain size-dependent, composite rheology. In EGU General Assembly Conference Abstracts (Vol. 19, p. 17433).


