

Assistant Professor Juliane Dannberg, University of Florida.
In this week’s Geodynamics 101 post, Juliane Dannberg, Assistant Professor at the University of Florida, outlines the role of mantle melt generation and transport in geodynamics.
Mantle melting and magma transport are important influences on the dynamics and chemical evolution of the Earth’s interior. All of Earth’s oceanic crust and depleted oceanic lithosphere is generated through melting at mid-ocean ridges, the main process to introduce chemical heterogeneity into the mantle. Mechanically, melt and fluids play an important role in the dynamics of plate boundaries like mid-ocean ridges and subduction zones, and in the formation of oceanic islands. Specifically, partially molten regions are weaker than solid rock, and because of that, mechanisms that localise melt often also localise deformation.
Many mantle convection models include the effects of melting and melt transport to a first order. They allow for the generation of oceanic crust and lithosphere when mantle material approaches the surface. This is typically done by using a thermodynamic model to determine where melting occurs, removing melt as soon as it it forms, and distributing it further up or at the surface of the model as basaltic crust. The residuum that is left behind becomes the depleted, harzburgitic lithosphere.
But considering the addition of melt, a low-viscosity fluid phase, in models of mantle dynamics is not only important for geochemical considerations. It also introduces new length and time scales and new dynamics. Rather than talking about numerical methods, my goal for this article is to give a short overview of the physics of magma dynamics, and to build intuition on how magma generation and transport can affect geodynamic processes.
The forces at play
In mantle convection models, the driving force, buoyancy, is usually balanced by viscous stresses.
Consequently, the length scales of convective features are usually determined by how fast boundary layers can grow, by the density differences causing material to be buoyant, and by the mantle viscosity. When fluids are added to this system, new forces become important1:
(1) Darcy drag. Melt can segregate from where it forms by flowing through the pore spaces of the solid host rock. We can think of the system as a sponge-like solid rock matrix that can be squeezed and stretched, saturated with liquid melt. As melt starts to flow, the traction on the interface between the melt and the mineral grains opposes this motion. The more pore space there is, the better it is connected, and the lower the viscosity of the melt, the easier it becomes for the for the melt to flow through.
(2) Viscous compaction. When melt is generated, or when melt is about to flow into a space that had no melt before, the pore space of the solid rock has to dilate so that melt can flow in. In other words, magma is pushing the mineral grains of the rock apart. Conversely, when melt flows out of an existing pore space, it pulls the grains together and the solid rock needs to compact to fill this volume. This means that the forces that drive the flow of melt have to overcome the resistance of the solid rock to volumetric deformation, governed by the host rock’s compaction viscosity.
The equations
So instead of the Stokes equation used in mantle convection models, magma dynamics is described by a more complicated force balance with additional terms (McKenzie, 1984; Scott & Stevenson, 1984,1986; Bercovici, Ricard et al., 2001, 2003; Sramek et al, 2007):
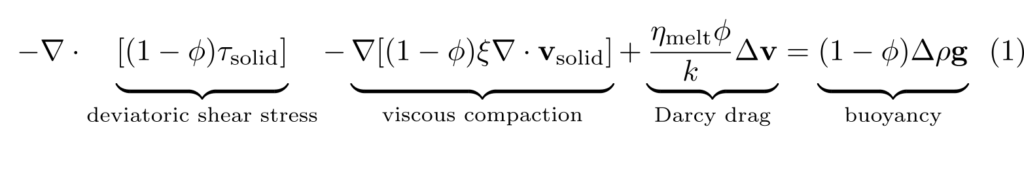
This equation includes a number of material properties that control magma dynamics. The melt viscosity ηmelt and the permeability k express how easy it is for melt to flow through the pore space of the rock. The larger the pore space, and the better the connection between the pores, the larger the permeability. Because the rock is saturated with melt, the amount of pore space, or porosity ϕ, is equivalent to the fraction of melt. Consequently, the more melt is present, the larger the permeability. Δv is the difference between solid velocity and melt velocity and can be expressed using Darcy’s law:
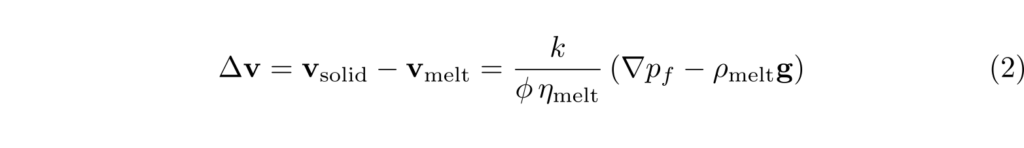
In words, melt segregation relative to the motion of the solid is driven by differences in the melt or fluid pressure pf relative to the hydrostatic pressure in the melt, ρmelt g.
The viscous stresses are controlled by the shear viscosity η and the compaction viscosity ξ. Both properties depend on the melt fraction: The more melt is present (or, the larger the pore space of the rock), the easier it becomes to deform the solid rock matrix. This dependence is quite strong, and as soon as the porosity reaches 20–30% (the disaggregation threshold), the solid rock will break apart and will no longer form a connected matrix. Instead, the mineral grains will be suspended within the melt. Buoyancy is controlled by the density difference between solid and melt Δρ and the gravity g.
Depending on the importance of each of these forces in a given setting, the transport of melt can occur in different ways (Sramek et al., 2007). Buoyancy is caused by the lower density of the melt compared to the solid rock, so the more melt is present, the stronger the buoyancy forces. If viscous compaction forces are small (which corresponds to rocks that are easy to deform), buoyancy is balanced by Darcy drag (the so-called Darcy equilibrium; Sramek et al., 2007). In this case, melt ascends pervasively through the pores of the host rock, and its segregation velocity is limited by the rock’s permeability. Accordingly, the more melt is present, the faster it can ascend. This mode of melt transport may be representative of melting zones below mid-ocean ridges, where the amount of melt present in the pores of the host rock is small.
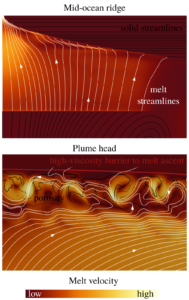
Figure 1: Below mid-ocean ridges, melt can segregate from the solid and flow towards the ridge axis (top, modified from Dannberg et al., 2019). If there is a high-viscosity barrier to melt ascent, such as at the top of plumes, melt may circulate together with the solid in form of diapirs (bottom).
Conversely, if Darcy drag is small, then buoyancy is balanced by viscous compaction (the so-called viscogravitational equilibrium; Sramek et al., 2007). Melt can only flow if the solid rock deforms, and in this case the viscosity of the rock limits how fast melt can rise. If the compaction viscosity is comparable to or smaller than the shear viscosity, the host rock expands and melt can flow through the pores (Scott, 1988). But if the bulk viscosity is much larger than the shear viscosity, melt is forced to stay in the pocket of host rock it was created in over a long time scale. Instead of segregating, it will ascend together with the solid in form of a diapir (Scott, 1988). Because the Darcy drag becomes smaller the more melt is present (corresponding to a larger permeability), this mode of melt transport may occur near the top of plume heads (Fig. 1; Dannberg & Heister, 2016). Within the plume, high temperatures reduce the viscosity, but above the plume head, ambient mantle viscosities are larger and may limit melt segregation.
The equations also introduce a new length scale that controls the size of features that emerge in magma dynamics. This scale is controlled by the ratio of viscous forces and Darcy forces:
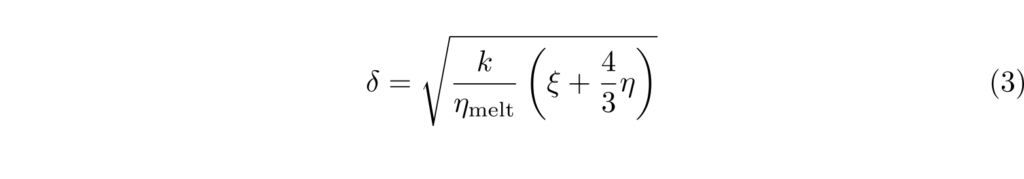
and is called the compaction length. It controls how far dynamic pressure differences between melt and solid, the compaction pressures, are transferred in partially molten rocks. This is important for the buoyant ascent of melt, which is hindered by the viscous resistance of the solid rock matrix to this compaction (Spiegelman 1993a, Spiegelman 1993b, Katz, 2015).
Solitary waves
Variations in porosity in the direction of gravity cause disturbances in the compaction pressure (Fig. 2): For example, if the porosity decreases in the direction of flow, the corresponding decrease in permeability makes it more difficult for melt to flow through and causes a decreasing flux of melt upwards into the low-porosity region. This negative gradient in melt flux leads to melt pressures being larger than solid pressures (a positive compaction pressure) at the location of the porosity perturbation, pushing the grains of the solid rock apart. This means that more melt can flow into this region and porosity increases. At the upper end of this perturbation, this same process continues to act, drawing more melt in.
At the lower end of the high-porosity region, the opposite happens: Because permeability increases in the direction of flow, the melt flux increases in upwards direction, causing a negative compaction pressure. Mineral grains are pulled together, and melt is expelled. Because the process continues in upwards and downwards direction, variations in the amount of melt will develop into magmatic waves (or solitary waves). At the front of the wave, the positive compaction pressure draws in more melt, and at the back of the wave, the negative compaction pressure pulls the mineral grains together as melt flows out. The length scale of these waves is on the order of the compaction length. In one dimension, this process can also be illustrated by the flow of fluid through a viscously deformable pipe (Scott, 1988). Imagine a part of the pipe that locally has a larger radius than the rest of the pipe and that moves upwards. To accommodate the arrival of more liquid, the pipe has to expand in front of the perturbation. In the same way, the pipe contracts behind the perturbation, reverting to its original radius.
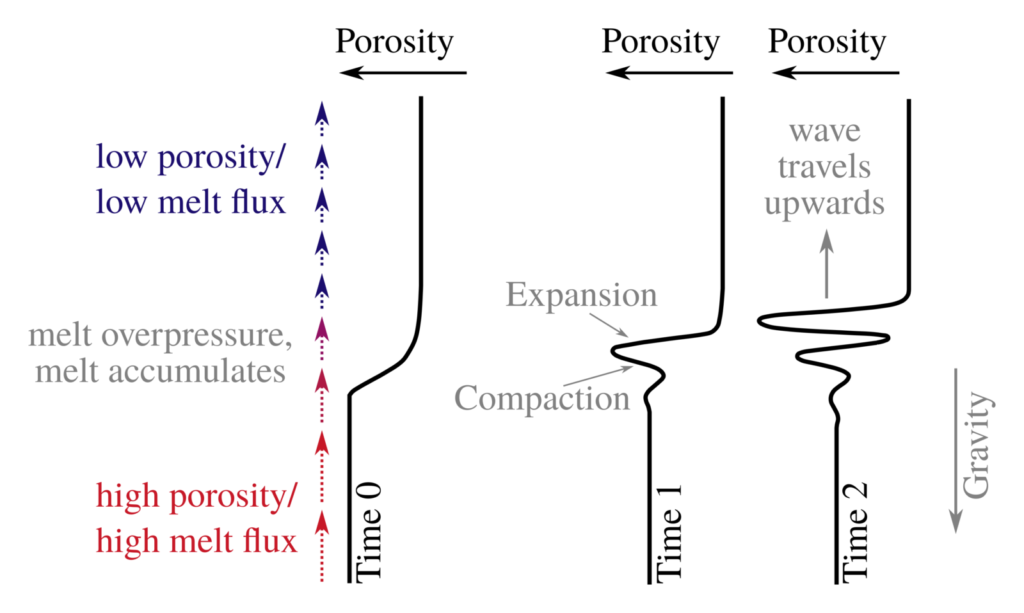
Figure 2: [Updated] Formation of solitary waves. After Spiegelman 1993b.
There are many more mechanisms that can cause melt to localise. When partially molten rock is sheared, melt is drawn into thin, melt-rich bands in between larger melt-poor regions. Channels of high porosity can form when reactive melting is driven by the flux of magma along a solubility gradient. A good overview over these processes and sources of further information is given in the lectures notes by Katz, 2015.
The bigger picture
All of these processes may be important for the flow of melt in subduction zones, at mid-ocean ridges, and for hotspot volcanism. Therefore I believe it is essential to better understand magma dynamics if we want to answer questions such as: How much of the mantle melt reaches the surface? What is the reason for the location and spacing of volcanoes? How are plate boundaries generated and maintained? I hope this article helps to understand this topic better and inspires you to consider melt generation and transport in this bigger context.
1These are the two most important forces in the mantle, but there are other forces that may impact deformation of partially molten rock. I have made a number of assumptions here, for example that the melt viscosity is much smaller than the solid velocity, that surface tension is negligible, and that deformation of the solid rock is predominantly viscous.
References: Bercovici, D. et al., 2001. A two‐phase model for compaction and damage: 1. General theory. J. Geophys. Res. Solid Earth, 106(B5), pp.8887-8906. Bercovici, D. and Ricard, Y., 2003. Energetics of a two-phase model of lithospheric damage, shear localization and plate-boundary formation. Geophys. J. Int., 152(3), pp.581-596. Dannberg, J. and Heister, T., 2016. Compressible magma/mantle dynamics: 3-D, adaptive simulations in ASPECT. Geophys. J. Int., 207(3), pp.1343-1366. Dannberg, J. et al., 2019. A new formulation for coupled magma/mantle dynamics. Geophys. J. Int., 219(1), pp.94-107. Katz, R.F., 2015. An introduction to coupled magma/mantle dynamics. http://foalab.earth.ox.ac.uk/files/IntroMagmaLectures.pdf McKenzie, D., 1984. The generation and compaction of partially molten rock. J. Petrol., 25(3), pp.713-765. Scott, D.R. and Stevenson, D.J., 1984. Magma solitons. Geophys. Res. Lett., 11(11), pp.1161-1164. Scott, D.R. and Stevenson, D.J., 1986. Magma ascent by porous flow. J. Geophys. Res. Solid Earth, 91(B9), pp.9283-9296. Scott, D.R., 1988. The competition between percolation and circulation in a deformable porous medium. J. Geophys. Res. Solid Earth, 93(B6), pp.6451-6462. Spiegelman, M., 1993a. Flow in deformable porous media. Part 1 Simple analysis. J. Fluid Mech., 247, pp.17-38. Spiegelman, M., 1993b. Flow in deformable porous media. Part 2 numerical analysis–the relationship between shock waves and solitary waves. J. Fluid Mech., 247, pp.39-63. Šrámek, O. et al., 2007. Simultaneous melting and compaction in deformable two-phase media. Geophys. J. Int., 168(3), pp.964-982.




Patrick Sanan
Thanks so much for this extremely useful post – it’s great to have a concise place to start with this topic!
(Trivial note: I think that the “porosity” axes in Figure 2 should point to the left)
Juliane Dannberg
Thank you for catching this! You are completely right about that.
George Bergantz
This is a wonderfully concise and compact review of what is assumed about melt transport, largely in the mantle domain and is appropriate there. But in the crust there is little direct evidence from petrography or outcrop for compaction, as described here and modeled by the equations of “viscous” processes, as an important means of melt extraction. So to the degree that one seeks to understand the processes that manifest the profound compositional diversity of Earth’s crust or the framework for volcanic processes, or volatile transfer, mathematical creations like solitary waves and postulates like viscous compaction must be critically evaluated by their verisimilitude with manifest observations where a non-continuum hydrogranular physics seems to be the norm.
Juliane Dannberg
That’s right, I only discuss magma dynamics in the parts of the mantle that are dominated by viscous deformation. But there is a great article on melt ascent through the lithosphere and crust by Tobias Keller and co-authors (https://doi.org/10.1093/gji/ggt306), which assumes a visco-elasto-plastic rheology and includes processes like hydrofracturing.