GD
Geodynamics

edited by Yinuo Zhang

Yinuo Zhang is a PhD student in marine geology at the University of the Chinese Academy of Sciences. She is interested in mid-ocean ridges, as well as their adjacent hotspots and transform faults. Her research focuses on analyzing geophysical data and geodynamical models to investigate the interactions among these geological features. Currently, she is working on the evolution of transform faults at the GEOMAR Helmholtz Ocean Research Centre, supported by the DAAD-CAS scholarship program.

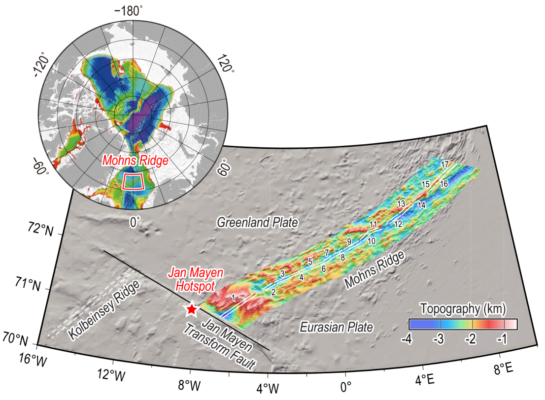
What’s the role of hotspot and oceanic transform faults at ultraslow spreading ridge?

and October 4, 2024
What’s the role of hotspot and oceanic transform faults at ultraslow spreading ridge?
Figure 1. Topographic map of the Mohns Ridge. The white double lines denote the axis of 17 ridge segments. (modified from Zhang et al., 2023)  

  Mid-ocean ridges (MOR) and hotspots are two types of magmatic activity occurring in the ocean. The MORs are typically associated with another tectonic feature—oceanic transform faults. While numerous studies have focused on the interactions within MORs, hotspots, and transform faults, there has been limited research on cases where a hotspot and a transform fault are located at the same end ...[Read More]

Seriously, science?

December 9, 2020
Seriously, science?

This week, Cédric Thieulot, Assistant Professor at The Department of Earth Science, in Utrecht, shows that there is no place for humor in peer reviewed scientific publications. Science is serious. It’s about experiments, theory, analysis, and for most of this readership maths, physics, chemistry, biology, geology and so much more. It’s serious business, with funding, promotion, titles, grants and ...[Read More]

And the solution to your lock-down-related sadness is…

October 21, 2020
Phat Thai!
A delicious Phat Thai, source: wikipedia. Mmmh look at this!

Cooking of course! Let me explain. This week, instead of doing his job, Antoine Rozel (senior researcher in ETH Zürich), shows that all your life problems will instantly disappear if you start cooking. Warning, this introductory paragraph is not going to cheer you up, skip it if you are already feeling blue unless you want to suffer a little more (that’s OK). Clearly the explosion of number ...[Read More]